面试题68 - I:二叉搜索树的最近公共祖先(C++)
2021-01-24 22:13
标签:节点 输入 面试 return nbsp com 源码 margin mamicode 题目地址:https://leetcode-cn.com/problems/er-cha-sou-suo-shu-de-zui-jin-gong-gong-zu-xian-lcof/ 给定一个二叉搜索树, 找到该树中两个指定节点的最近公共祖先。 百度百科中最近公共祖先的定义为:“对于有根树 T 的两个结点 p、q,最近公共祖先表示为一个结点 x,满足 x 是 p、q 的祖先且 x 的深度尽可能大(一个节点也可以是它自己的祖先)。” 例如,给定如下二叉搜索树: root = [6,2,8,0,4,7,9,null,null,3,5] 示例 1: 输入: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 8 输入: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 4 说明: 所有节点的值都是唯一的。 要理解最近公共祖先,首先要明确祖先的定义,若一个节点cur在另一个节点prev的左右子树,或者节点cur等于节点prev,则节点prev就是节点cur的祖先节点。而最近公共祖先可以表示成,我们假设root是节点p和q的公共祖先节点,如果root的左节点left和右节点right都不是p和q的公共祖先,则root就是节点p和q的最近公共祖先节点。具体来说,如果节点root为最近公共祖先节点,只能是以下几种情况 另外,观察题目给出的树为二叉搜索树以及条件说明,我们可以得出如下的结论 非递归 递归 参考文章 https://leetcode-cn.com/problems/er-cha-sou-suo-shu-de-zui-jin-gong-gong-zu-xian-lcof/solution/mian-shi-ti-68-i-er-cha-sou-suo-shu-de-zui-jin-g-7/ (动画清晰明了) 面试题68 - I:二叉搜索树的最近公共祖先(C++) 标签:节点 输入 面试 return nbsp com 源码 margin mamicode 原文地址:https://www.cnblogs.com/wzw0625/p/12863763.html题目描述
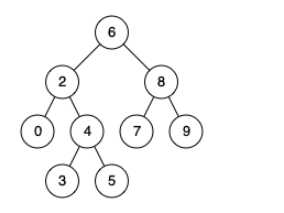
题目示例
输出: 6
解释: 节点 2 和节点 8 的最近公共祖先是 6。
示例 2:
输出: 2
解释: 节点 2 和节点 4 的最近公共祖先是 2, 因为根据定义最近公共祖先节点可以为节点本身。
p、q 为不同节点且均存在于给定的二叉搜索树中。解题思路
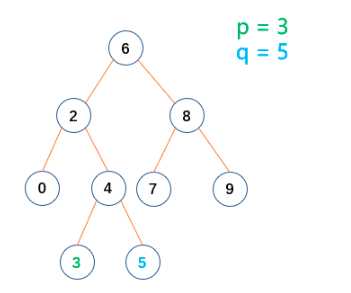
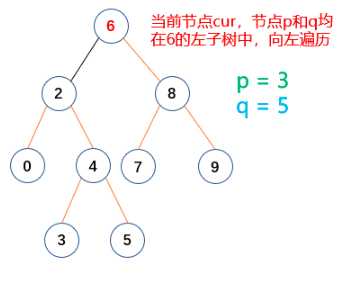
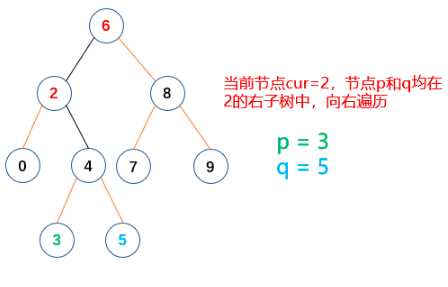
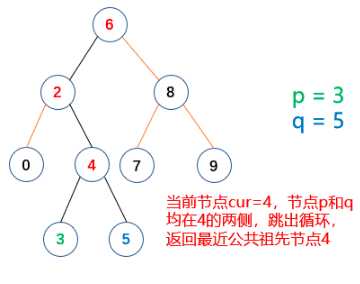
程序源码
/**
* Definition for a binary tree node.
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode(int x) : val(x), left(NULL), right(NULL) {}
* };
*/
class Solution {
public:
TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {
TreeNode* cur = root;
while(cur != nullptr)
{
if(cur->val > p->val && cur->val > q->val)
{
cur = cur->left; //节点p和节点q均在节点cur的左子树中,继续向左遍历
}
else if(cur->val val && cur->val val)
{
cur = cur->right; //节点p和节点q均在节点cur的右子树中,继续向右遍历
}
else
break; //节点p和节点q均在节点cur的两侧,跳出循环,找到了最近公共祖先节点cur
}
return cur;
}
};
/**
* Definition for a binary tree node.
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode(int x) : val(x), left(NULL), right(NULL) {}
* };
*/
class Solution {
public:
TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {
if(root == nullptr) return nullptr;
if(root->val > p->val && root->val > q->val)
{
return lowestCommonAncestor(root->left, p, q);
}
if(root->val val && root->val val)
{
return lowestCommonAncestor(root->right, p, q);
}
return root;
}
};
上一篇:使用python获取当前的时间
下一篇:js 一维数组转二维数组