数据结构与算法-绪论

标签：return   问题   例子   可行性   滚动数组   斐波那契数   空间   str1   状态   

绪论

算法：即是在特定计算模型下，旨在解决特定问题的指令序列
要保证正确性、确定性、可行性、有穷性
有穷性：

例子1：HailStone序列

 @Test
    public void test1() {

        int n = 7;
        int length = 1;
        while (n > 1) {
            n = ((n % 2) > 0) ? 3 * n + 1 : n / 2;
            length++;
        }
        System.out.println(length);
    }

层级级别：

例子2：计算任意N个整数之和

减而治之

@Test
    public void test4() {
        int[] A = {100, 836, 3236, 5, 16, 26, -3, 89, 69, 43};

        long begintime = System.nanoTime();
        /*int n = A.length;//简单递归
        System.out.println(sum1(A,n));*/
        int lo = 0;//二分递归
        int hi = A.length - 1;
        System.out.println(sum2(A, lo, hi));
        long endtime = System.nanoTime();
        long costTime = (endtime - begintime);
        System.out.println(costTime);

    }

    public int sum1(int A[], int n) {
        return (n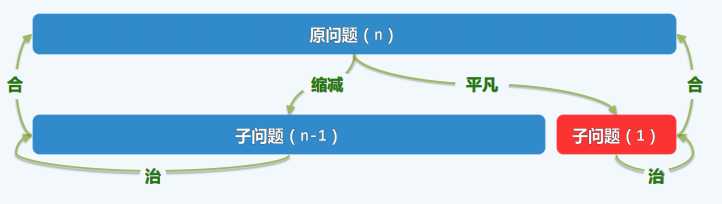 

分而治之

public int sum2(int A[], int lo, int hi) {
        if (lo == hi) {
            return A[lo];
        }
        int mi = (lo + hi) >> 1;
        return sum2(A, lo, mi) + sum2(A, mi + 1, hi);
    }

数组反向

@Test
    public void test5() {
        int[] A = {100, 836, 3236, 5, 16, 26, -3, 89, 69, 43};
        int lo = 0;
        int hi = A.length - 1;
        reverse(A, lo, hi);
    }

    public void reverse(int[] A, int lo, int hi) {
        if (lo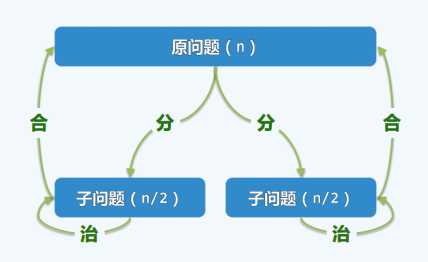 

例子3：从数组区间中找出最大的两个整数元素

@Test
    public void test6() {
        int[] A = {100, 836, 3236, 5, 16, 26, -3, 89, 69, 43};
        int lo = 0;
        int hi = A.length - 1;
        /*int[] int1 = max2(A, lo, hi);*/
        /*int[] int2 = max02(A, lo, hi);*/
        int[] int3 = max002(A, lo, hi);

        System.out.println(Arrays.toString(int3));
    }

    public int[] max2(int[] A, int lo, int hi) {
        int x1 = lo;
        int x2 = lo;
        //扫描lo-hi,找到x1
        for (int i = lo + 1; i 

改进1：

public int[] max02(int[] A, int lo, int hi) {
        int x1 = lo;
        int x2 = lo + 1;
        if (A[x1] 

改进2：

public int[] max002(int[] A, int lo, int hi) {
        int x1 = 0;
        int x2 = 0;
        if (lo + 1 == hi){
            if (A[x1 = lo]  A[mid]) && (A[lo] > A[hi])){
                x1 = lo;
                x2 = (A[mid] > A[hi]) ? mid:hi;
            }else if((A[lo]  A[hi])){
                x1 = mid;
                x2 = (A[lo] > A[hi]) ? lo:hi;
            }else{
                x1 = hi;
                x2 = (A[lo] > A[mid]) ? lo:mid;
            }
            return new int[]{x1,x2};
        }

        int mi = (lo + hi) / 2;
        int[] L= max002(A, lo, mi);
        int[] R = max002(A, mi+1, hi);

        if (A[L[0]] > A[R[0]]){
            x1 = L[0];
            x2 = (A[L[1]] > A[R[0]]) ? L[1]:R[0];
        }else{
            x1 = R[0];
            x2 = (A[L[0]] > A[R[1]]) ? L[0]:R[1];
        }
        return new int[]{x1,x2};
    }

相关链接

例子3.5：最大综合区间（还没写）

蛮力

递增

分治

减治

例子4：斐波那契数列

动态规划

@Test
    public void test7(){
        for (int i = 0;i 

例子5：最长公共子序列

情况分析

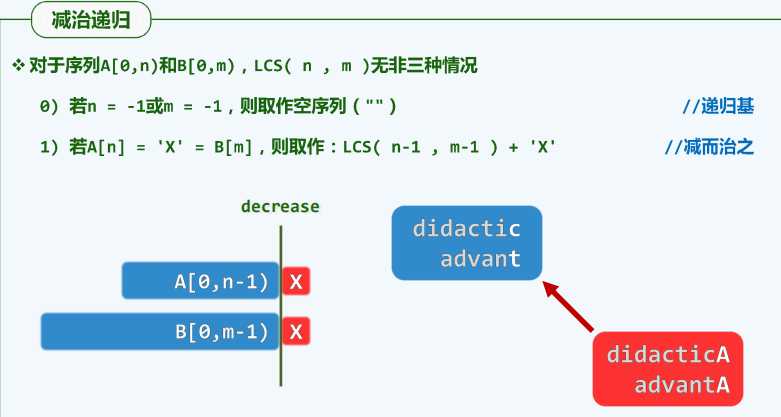
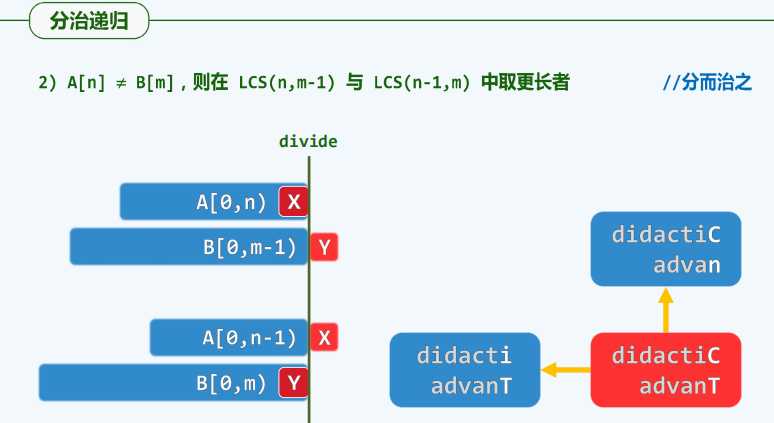
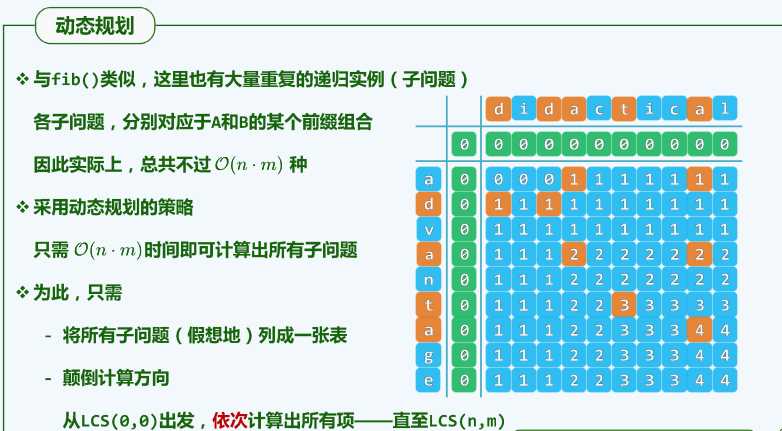
@Test
    public void test8(){
        char[] x = {‘A‘,‘B‘,‘C‘,‘B‘,‘D‘,‘A‘,‘B‘};
        char[] y = {‘B‘,‘D‘,‘C‘,‘A‘,‘B‘,‘A‘};
        int[][] b = new int[x.length+1][y.length+1];
        int[][] c = lcsLength(x,y,b);
        System.out.println(c[x.length][y.length]);
        lcs(x.length,y.length,x,b);
    }
    //从[0][0]向[x.length+1][y.length+1]不断的得到1、共同序列个数 2、各种情况并作出标记
    public int[][] lcsLength(char[] x,char[] y,int[][] b) {
        //给第一行，第一列设置空序列
        int[][] c = new int[x.length+1][y.length+1]; //0存空序列
        for(int i=0;i=c[i][j-1]){
                        c[i][j] = c[i-1][j];
                        b[i][j] = 2;
                    }else{
                        c[i][j] = c[i][j-1];
                        b[i][j] = 3;
                    }
                }
            }
        }
        //返回规划好的情况c
        return c;
    }
    public void lcs(int i,int j,char[]x,int[][]b){
        //结束条件
        if (i==0 || j==0){
            return;
        }
        //判断b[i][j]进入不同分支
        if (b[i][j]==1){
            //减而治之
            lcs(i-1,j-1,x,b);
            System.out.print(x[i-1]);
        }else {
            //分而治之
            if (b[i][j] == 2){
                lcs(i-1,j,x,b);
            }else{
                lcs(i,j-1,x,b);
            }
        }
    }

空间优化

/**
     * 方法二、进行空间上的优化
     * 通过状态方程可知，计算c[i][j]时只需知道c[i-1][j-1]、c[i-1][j]、c[i][j-1]就行了
     * 那么就和斐波那契数列比较相似，可以利用滚动数组
     */
    //从[0][0]向[x.length+1][y.length+1]不断的得到1、共同序列个数 2、各种情况并作出标记
    public Set lcsLength2(char[] x, char[] y) {
        Set set = new HashSet();
        //给第一行，第一列设置空序列
        int[][] c = new int[x.length+1][y.length+1]; //0存空序列
        for(int i=0;i

递归版本（没写JAVA版的）

function LCS(str1, str2, a, b) {
      if(a === void 0){
          a = str1.length - 1
      }
      if(b === void 0){
          b = str2.length - 1
      }
      if(a == -1 || b == -1){
          return 0
      } 
      if(str1[a] == str2[b]) {
         return LCS(str1, str2,  a-1, b-1)+1;
      }
      if(str1[a] != str2[b]) {
         var x =  LCS(str1, str2, a, b-1)
         var y =  LCS(str1, str2, a-1, b)
         return x >= y ? x : y
      }
  }

相关链接

例子6：就地循环位移（还没写）

例子7：就地随机置乱（还没写）

数据结构与算法-绪论

标签：return   问题   例子   可行性   滚动数组   斐波那契数   空间   str1   状态   

原文地址：https://www.cnblogs.com/suit000001/p/13373802.html